LECTURE 7

Chemical Bonding: Ionic, Covalent, and Metallic

When atoms bond chemically, they form molecules and solids. The electrons in the outermost shells of an atom are called valence electrons and these are the electrons which are responsible for chemical bonding. The valence electrons are the ones outside the filled shells.

Ionic bonding is characterized by electron transfer; one of the reacting atoms loses one or more electrons, and the other atom gains one or more electrons. For example, consider sodium chloride (NaCl, otherwise known as table salt). Sodium is an alkali metal and chlorine is a halogen. To get a complete shell, a sodium atom gives its outer 3selectron to a chlorine atom which otherwise would be one electron short of a complete shell. As a result the sodium ion (Na⁺) acquires a 1+ charge and the chlorine ion (Cl^-) acquires a 1- charge. Positive ions are called cations and negative ions are called anions. We say that chlorine is very electronegative. Electronegativity is a measure of the ability of an atom in a molecule to attract (or hog) electrons to itself.

In covalent bonding electrons are not transferred but are shared; a covalent bond consists of a pair of electrons (with opposite spins in a singlet state) shared by two atoms. An example is the covalent bond formed by two hydrogen atoms in an H₂ molecule. The electrons are attracted by the positive nuclear charge to the region between the nuclei. The 1s orbitals overlap to form a sigma bonding orbital ($\sigma$). The spatial part of the sigma bonding orbital $\psi_{\sigma}$ is a symmetric linear combination of the 1s orbitals:

$$\psi_{\sigma}=\frac{1}{\sqrt{2}}(\psi_{s,A}+\psi_{s,B})$$ (1)

where A denotes one atom and B denotes the other atom. The sigma bonding orbital has a lower energy than either of the 1s orbitals because of the Coulomb attraction between the electrons and the nuclei. So it is energetically favorable to form the covalent bond. The electrons in a sigma bond are in a spin singlet state.

There is also a sigma antibonding orbital ( $\sigma^{*}$) which is spatially an antisymmetric linear combination of the 1s orbitals:

$$\psi_{\sigma^{*}}=\frac{1}{\sqrt{2}}(\psi_{s,A}-\psi_{s,B})$$ (2)

This is a higher energy orbital in which the electron density between the nuclei is depleted. The energy of the antibonding orbital is higher than the energy of either 1s atomic orbital, so it is not energetically favorable to put electrons in the antibonding orbital. The electrons in a sigma antibonding orbital are in a spin triplet state.

Notice that the number of single electron states is conserved in bonding. We started with 2 individual atoms, each with a 1s orbital. That makes a total of 4 single electron states, counting spin. Covalent bonding leads to 2 sigma orbitals ($\sigma 1s$ and $\sigma^{*}1s$) which have a total of 4 single particle states. If we try to form a helium molecule, He₂, we would put the two 1s orbitals together and form the $\sigma 1s$ and $\sigma^{*}1s$orbitals. Then we would fill them with the 4 helium electrons. The energy gained by putting two electrons in the sigma bonding orbital is lost by putting the other two electrons in the sigma antibonding orbital. So He₂ is unstable and no bond is formed. Helium is a noble gas and doesn't need other electrons to complete its atomic orbital shell.

The molecular orbitals derived from the overlap of 2p atomic orbitals are slightly more complicated. The three 2p orbitals of an atom are directed along the Cartesian coordinates x, y, and z. If we consider that a diatomic molecule is formed by the atoms approaching each other along the x axis, the p_x atomic orbitals approach each other head on and overlap to produce $\sigma 2p$ bonding and $\sigma^{*}2p$antibonding molecular orbitals. All sigma orbitals are symmetrical about the internuclear axis; the $\sigma 2p$ and $\sigma^{*}2p$orbitals have shapes that are similar to those of the $\sigma 1s$and $\sigma^{*}1s$ orbitals.

In the formation of a diatomic molecule, the p_z atomic orbitals approach each other side to side and produce pi ($\pi$) bonding and antibonding molecular orbitals. $\pi$ orbitals are not symmetrical about the internuclear axis. The electron density of a $\pi$ bonding orbital is zero in a plane that includes both nuclei of the molecule, but charge is concentrated in two regions that lie above and below this plane and between the two nuclei. The $\pi^{*}$ orbital reduces electronic density in the internuclear region.

The p_y orbitals also approach each other sideways. Consequently another set of $\pi$ and $\pi^{*}$ molecular orbitals, which lie at right angles to the set resulting from the overlap of p_zorbitals, can be produced. The two $\pi 2p$ orbitals are degenerate (have equal energy), and the two $\pi^{*} 2p$ orbitals are degenerate. Six molecular orbitals, therefore, arise from the two sets of porbitals-one $\sigma 2p$, one $\sigma^{*}2p$, two $\pi 2p$, and two $\pi^{*} 2p$.

In forming a diatomic molecule, the $\sigma2s$ and $\sigma^{*} 2s$ orbitals are lower in energy than the orbitals formed from the 2p orbitals. So the $\sigma2s$ and $\sigma^{*} 2s$ orbitals will be occupied before the $\sigma 2p$ or $\pi$ orbitals are.

Pure ionic bonding and pure covalent bonding are seldom encountered. Most bonds have intermediate character, although many bonds are predominantly either ionic or covalent. In heteronuclear, diatomic molecules the clouds of the bonding orbitals are distorted so that more electronic charge is located around the more electronegative atom than around the other atom; antibonding orbitals have their largest electron density in the regions close to the less electronegative atom. The molecular orbital wavefunction has the form

$$\psi_{MO}=c\psi_A+d\psi_B$$ (3)

In some molecules which consist of more than 2 atoms, there can be multicenter or delocalized bonding in which some of the bonding electrons bond more than 2 atoms. One example is the $\pi$ bonding found in benzene which consists of 6 carbon atoms in a ring. A carbon atom has 4 valence electrons, so it wants to form 4 bonds to have a filled shell. In benzene each carbon atom forms one bond with a hydrogen atom and 3 bonds with its neighboring 2 carbon atoms.

The ultimate in delocalized bonding is metallic bonding. In a metal the atoms contribute their valence electrons to the collective whole. As a result the electrons do not belong to any specific atom, but have wavefunctions that extend throughout the whole system. These conduction electrons are responsible for holding the metal together. This is metallic bonding.

Nuclear Physics

From the viewpoint of nuclear physics, the nucleus consists protons and neutrons which are called nucleons. The total number A of nucleons in the nucleus is called the mass number. The nuclear mass is approximately A times the mass of a hydrogen atom. The number of protons is denoted by Z and is called the atomic number. The atomic number gives the charge of the nucleus and determines which element the atom belongs to. Different isotopes of a given element all have the atomic number Z but different numbers of neutrons, i.e, different A. The radius of a nucleus is about 1.2A^1/3 fermi, where a fermi (F) is 10^-15 meters. (The 1/3 power makes sense since the volume of a nucleus goes as the number A of nucleons.) So a nucleus is typically a few fermi ( $\stackrel{<}{\sim}$ 10 F). To get some idea of the scale, if an atom had a radius of 1 km, the nucleus would have a radius of order a few cm. The nucleons are held together by the strong force which is a short range interaction between the nucleons. The range of the interaction is about 2 F and is attractive. At that range the strong force is 100 times larger than the Coulomb force. The strong force is independent of electric charge, i.e., it is the same between protons and neutrons as between protons and protons.

I want to mention 2 models of the nucleus. One is called the liquid drop model and the other is called the nuclear shell model. The liquid drop model is based on two properties that are common to all except the lightest nuclei, (1) the interior mass densities are approximately the same and (2) the binding energy per nucleon is approximately 8 MeV. The liquid droplet model views the nucleus as a tiny incompressible drop of liquid with the constituents being nucleons. In physics a ``gas'' is used to describe a bunch of noninteracting particles whose energy consists only of kinetic energy, and a liquid is a ``gas'' of interacting particles. The liquid droplet model views the nucleons largely as classical particles.

Nuclear Shell Model

One of the major shortcomings of the liquid droplet model is that it cannot explain the ``magic numbers.'' Let N be the number of neutrons in the nucleus. Nuclei with certain values of Z and/or N are unusually stable. These values of Z and/or N are called the magic numbers:

$$Z\;{\rm and/or}\;N=2,8,20,28,50,82, 126$$ (4)

Nuclei prefer to have these values of Z and/or N. The evidence for stability comes from the fact that there are more than the average number of stable isotopes with these magic numbers. For example there are 10 stable isotopes with Z=50whereas nuclei in that vicinity of Z typically have about 4 stable isotopes. Another piece of evidence is the anomalously large binding energy of the ``last'' neutron or proton making up a magic number. The magic numbers are reminiscent of the chemical inertness of the noble gas atoms that have a closed shell of electrons. The nuclear shell model treats the nucleons as fermions complete with the Pauli exclusion principle. The protons and neutrons occupy energy levels which are similar to the electronic energy levels found in an atom. In the shell model the nucleons are assumed to move in a potential that is a sum of a harmonic oscillator potential, a square well potential, and spin-orbit coupling. The harmonic oscillator potential and the square well are radial in the sense that they are functions only of the radial coordinate (V(r)). The 3D harmonic oscillator energy levels are given by

$$E_{HO}=\left(N+\frac{3}{2}\right)\hbar\omega$$ (5)

where $N=n_x+n_y+n_z=2(n_r-1)+\ell$ and (n_r-1) is the number of radial nodes in the wavefunction.

$$\hbar\omega=\frac{41}{A^{1/3}}\;{\rm MeV}$$ (6)

Spin-orbit coupling splits the $j=\ell\pm 1/2$ levels. The energy levels of the shell model are shown in Figure 15-18 of Eisberg and Resnick. The half integers are the j values of the states. Note that significant gaps lie above each of the levels marked with a magic number in the last column. The gaps indicate the stability of the magic numbers.

Besides predicting the magic numbers, the shell model can also predict the total angular momentum I of the ground states of almost all the nuclei. (The total angular momentum of the nucleus is often referred to as its spin.) It turns out that pairs of nucleons of the same type (e.g., pairs of protons) strongly tend to combine with one another to yield zero angular momentum. Thus all even-even nuclei have I=0. ``Even-even nuclei'' means nuclei with even numbers of protons and even numbers of neutrons. Nucleons of the same kind tend to pair because it increases their overlap and enables them to take advantage of the short range attractive strong force. A simple example is the fact that 2 fermions in a singlet state, which is antisymmetric in spin space, have a symmetric spatial wavefunction which has more overlap than its antisymmetric spatial counterpart. Now consider the case of odd-A nuclei which have an even number of nucleons of one kind and an odd number of the other. All the nucleons pair to yield zero angular momentum except the odd nucleon. The angular momentum of the odd-A nucleus should just be that of the odd nucleon, and indeed this is the case for virtually all odd-A nuclei whose spin is known. Further the same argument applied to (presumably unstable) odd-odd nuclei suggests that their angular momentum ought to be some combination of the angular momenta of the two odd nucleons. Again this is observed to be the case, but, of course, this test is not nearly as definitive as the first one. Odd-odd nuclei have an odd number of neutrons and an odd number of protons. An odd-odd nucleus should be unstable because it can become an even-even nucleus of lower energy by having one of its neutrons decay into a proton. A neutron can decay into a proton, an electron, and an electron antineutrino in a process called beta decay.

$$n\rightarrow p + e^- + \bar{\nu}_e$$ (7)

This process is said to occur by the so-called ``weak interaction.'' There are 4 fundamental types of interactions: gravitational, electromagnetic, weak, and strong. We will talk more about this later when we discuss particle physics.

The shell model has also met with considerable success in providing a semiquantitative description of many of the properties of the low-lying excited states of most odd-A nuclei.

Another interesting phenomenon for which the shell model provides an illuminating semiquantitative explanation is that of nuclear isomerism. In the study of the excited states of nuclei it is found that certain nuclei can exist for quite extended periods of time in an excited state, from which they often finally decay by $\gamma$-ray emission. Such states may have decay lifetimes ranging up to many days. The nuclei which can exist in these so-called metastable states tend to be those having a nearly closed shell configuration of nucleons. The explanation of the long lifetimes of these metastable states lies in the fact that the ground state and the first excited state differ in angular momentum by several units of $\hbar$. Thus, many of the lower-order transitions, such as electric dipole, magnetic dipole, and electric quadrupole, are forbidden, so that a transition of very high multipole order may be required. The probability of transition rapidly becomes smaller with increasing multipole order, so that a long lifetime for the state results.

Another nuclear property for which the shell model provides an explanation is the parity of the ground state. Recall that parity refers to whether the spatial part of the wavefunction changes sign when $\vec{r}\rightarrow -\vec{r}$. By the arguments used in connection with angular momenta, the ground state parity of even-even nuclei should be even, and that of odd-A nuclei should correspond to $(-1)^{\ell}$ where $\ell$ is the orbital angular momentum of the odd nucleon. This rule is observed to lead to the correct parities for virtually all cases.

So we can see that the nuclear shell model is quite good at explaining many of the properties of nuclei.

Fission

Fission is the process by which a nucleus breaks into 2 or more pieces. This breakup may be spontaneous because the nucleus is unstable as in the radioactive isotopes, or it may be induced by bombardment of a particle such as a neutron. The fragments which result from a fission process are smaller nuclei which are usually radioactive. The idea that a nucleus can break up into smaller nuclei is quite natural in the context of the liquid drop model. This picture of fission was first pointed out by Lisa Meitner and her nephew, Otto Frisch in 1938. They were trying to understand some experiments in which Otto Hahn and F. Strassman found that bombarding uranium (Z=92) with neutrons produced radioactive barium (Z=56) among its products. Once this general idea was clear, comparison of the atomic weight of uranium with the sum of the weights of the fission products showed that a very large amount of energy would be set free in fission. Frisch immediately proved this experimentally. Further, the fraction of neutrons in the nucleus, N/A=(A-Z)/A, was much larger in uranium than in the fission products; hence neutrons would be set free in fission. This was proved experimentally by Joliot and Curie. Later experiments showed that the average number of neutrons per fission was 2.5. This opened the way for the chain reaction. In a chain reaction on average at least one of the neutrons released by fission of a U-235 nucleaus must be captured by another U-235 nucleus which then fissions. The first chain reaction was established by Enrico Fermi and his collaborators on December 2, 1942 at the University of Chicago. They used a ``pile'' of graphite bricks with a lattice of uranium metal inside. Controlled chain reactions powers nuclear reactors and explosive chain reactions are used in nuclear weapons. There are currently about 400 nuclear power plants in operation.

Niels Bohr and John Wheeler produced a general theory of fission in 1939. They predicted that only the rare isotope of uranium, U-235, would be fissionable by slow neutrons. The reason is that U-235 has an odd number of neutrons. The incoming neutron binds with the odd neutron to form a neutron pair and the energy gained from this binding (of order an MeV) helps the nucleus to deform and break apart. However, U-238 has an even number of neutrons and doesn't gain the extra binding energy from forming a pair. So the energy to deform and fission the U-238 nucleus comes from the kinetic energy of the incoming neutron. Nier showed experimentally that indeed U-235 can be fissioned by slow neutrons while U-238 requires neutrons of about 1 MeV. Since the nuclear cross section goes as 1/E where E is the energy of the incoming neutron, slower neutrons have a bigger chance of hitting a nucleus than faster neutrons. (The cross section $\sigma$ = decay rate/(incoming flux of particles).) So it's easier to cause fission in U-235 than in U-238. In nuclear reactors graphite is used to slow the fission neutrons, originally emitted at about 1 MeV energy, down to thermal energies which is less than 1 eV. Graphite is a form of carbon which absorbs very few neutrons. Heavy water works even better but is harder to come by. The heat from water used to cool the graphite is used to make steam which in turns runs turbines and generates electricity in a nuclear power plant. Neutron absorbers, like rods of boron, are used to control the reaction. The rods are inserted to slow the reaction and taken out if the reaction is too going to slowly.

NMR: Nuclear Magnetic Resonance

(Reference: C. P. Slichter, Principles of Magnetic Resonance, 3rd edition.)
NMR is an extremely powerful tool for probing the local properties of solids (and liquids). Principally it enables us to use the nucleus as a local probe to get information about solids, much as radioactive tracers are used in biological systems. Not only is it used in physics and chemistry, but it is also used in medicine where it is called MRI (Magnetic Resonance Imaging). For nuclei of nonzero spin the degeneracy of the energy levels with respect to the orientation of the nuclear spin is lifted by an applied magnetic field. This is analogous to the Zeeman effect for electronic levels. Resonant absorption of electromagnetic energy occurs when the photons bombarding the system have an energy equal to the energy level splitting.

To understand this in more detail, consider a set of identical nuclei of spin I and magnetic moment $\vec{\mu}=g_n\mu_n\vec{I}=\gamma\hbar\vec{I}$, where g_nis the dimensionless nuclear g-factor (typically $\sim 1$), $\mu_n=e\hbar/2M_Pc$ is the nuclear magneton, and $\gamma$is the gyromagnetic ratio ( $\gamma=g_n\mu_n/\hbar$). (In $\mu_n$ we just have the proton mass M_P rather than the mass of all the nucleons because it's usually the odd nucleon that determines $\vec{I}$.) We start with a simple quantum mechanical view of resonance theory. If we apply a magnetic field $\vec{H}$, the Hamiltonian is

$${\cal H}=-\vec{\mu}\cdot\vec{H}$$ (8)

Taking the field $\vec{H}=H_o\hat{z}$, we find

$${\cal H}=-\gamma\hbar H_o I_z$$ (9)

In the presence of the field, the energy levels are Zeeman split

$$E=-\gamma\hbar H_o m \;\;\;\; m=-I,-I+1,...,I-1,I$$ (10)

lec7levels.eps

We can get transitions between the levels by applying an alternating rf field perpendicular to the static field. (rf stands for radio frequency.) We get a transition if

$$\hbar\omega=\Delta E$$ (11)

where $\Delta E$ is the energy difference between initial and final nuclear states, and $\omega$ is the frequency of the rf field. The rf field consists of photons of frequency $\omega$. It turns out that the rf field induces transitions predominantly between adjacent levels, so that $\Delta E$ is the energy difference between adjacent levels

$$\hbar\omega=\Delta E=\gamma\hbar H_o$$ (12)

So

$$\omega=\gamma H_o$$ (13)

This is the resonance condition, i.e., the condition for resonant absorption of the rf photons by the nuclei.

To see why the transitions are between adjacent levels, notice that the rf field is given by

$$\vec{H}_{\rm rf}=H_x^o \cos \omega t \; \hat{x}$$ (14)

The Hamiltonian for the rf field is

$${\cal H}_{\rm rf}=-\vec{\mu}\cdot\vec{H}_{\rm rf}= -\gamma\hbar H_x^o I_x\cos \omega t$$ (15)

Recall that from Fermi's Golden Rule (lecture 6) that the transition rate induced by ${\cal H}_{\rm rf}$ is

$$R_{f\leftarrow i}=\frac{2\pi}{\hbar}\; \vert<m^{\prime}\vert{... ...rf}\vert m>\vert^2 \; \delta(E_{m^{\prime}}-E_m- \hbar \omega)$$ (16)

where m and $m^{\prime}$ are the magnetic quantum numbers of the Zeeman split states. It turns out that because I_x=(I⁺+I^-)/2

$$<m^{\prime}\vert{\cal H}_{\rm rf}\vert m>\sim\delta_{m^{\prime},m\pm 1}$$ (17)

So ${\cal H}_{\rm rf}$ connects adjacent Zeeman split levels.

Note that Planck's constant has disappeared from the resonance condition, implying that a classical picture could also be used. We will describe such a picture, but first, some numbers. Note that $\mu_B=e\hbar/2m_ec$and $\mu_N=e\hbar/2M_Pc$. Since $M_P/m_e\simeq 1800$, nuclear numbers differ from electronic numbers by about 10³. Thus $\gamma_e\sim10^{11}$Hz/Tesla and $\gamma_N\sim 10^7$ Hz/Tesla. Electronic systems have a resonance at $\sim 10,000$ MHz ( $\lambda \sim 3$ cm which is in the microwave region), whereas nuclear systems typically resonate at 10 MHz (a radio frequency) for $H_o\sim 3000$ to 10,000 Gauss.

Classical Treatment A magnetic moment $\vec{\mu}$ in a magnetic field $\vec{H}$ experiences a torque $\vec{\tau}=\vec{\mu}\times\vec{H}$. Thus

$$\vec{\tau}=\frac{d\vec{L}}{dt}=\hbar\frac{d\vec{I}}{dt}=\vec{\mu}\times\vec{H}$$ (18)

where $\vec{I}$ is the total angular momentum (also called spin) of the nucleus. Since $\vec{\mu}=\gamma\hbar\vec{I}$,

$$\frac{d\vec{\mu}}{dt}=\vec{\mu}\times\gamma\vec{H}$$ (19)

Physically we can think of the spin precessing about the field $\vec{H}$. This is Larmor precession. If $\vec{H}=H_o\hat{z}$, then the magnetic moment precesses at an angular frequency of $\omega=-\gamma H_o\hat{z}$. (Recall that $\vec{\tau}=\vec{\omega}\times\vec{L}$.) Now suppose we transform to the reference frame that is rotating with this angular frequency. In this rotating frame, $\vec{\mu}$is fixed and does not see the magnetic field $H_o\hat{z}$. (If it saw a magnetic field, it would precess.)

lec7precess.eps

Now let's examine the effect of the alternating magnetic field. $H_x(t)=H_{x}^o\cos\omega t$ is most readily analyzed by breaking it into two rotating components, each of amplitude H_ox, one rotating clockwise and the other counterclockwise. We denote the rotating fields by $\vec{H}_R$ and $\vec{H}_L$:

$$\vec{H}_x(t)=\vec{H}_R(t)+\vec{H}_L(t)$$ (20)

where

$$\begin{eqnarray*}\vec{H}_R(t)&=&\frac{1}{2}H_{x}^o(\hat{x}\cos\omega t+\hat{y}\s... ...=&\frac{1}{2}H_{x}^o(\hat{x}\cos\omega t-\hat{y}\sin\omega t)\\ \end{eqnarray*}$$

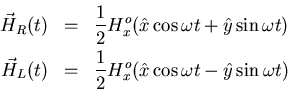
lec7Hx.eps

Let's suppose that $\omega=\gamma H_o$. This is the resonance condition. In the reference frame rotating with the magnetic moment of the nucleus, H_L looks like a static field pointing along the x axis, while H_R is rotating at $2\omega$ and averages to zero. So we can ignore H_R.

So in the rotating frame at resonance ( $\omega=\gamma H_o$), the magnetic moment sees a static magnetic field $\vec{H}_{1}=\frac{1}{2}H_{x}^o\hat{x}$pointing along the x axis. So if $\vec{\mu}$ initially points along the $\hat{z}$, then it will precess in the y-z plane about the x axis. If we turn on H₁ for a short time t_w, the moment precesses through an angle $\theta=\gamma H_{1}t_w$. If $\theta=\pi$, the pulse inverts $\vec{\mu}$. This is called a ``180 degree pulse'' or ``$\pi$ pulse.'' If $\theta=\pi/2$, $\vec{\mu}$ is rotated until it lies parallel to $\hat{y}$. This is called a ``90 degree pulse'' or a ``$\pi/2$ pulse.'' After H₁ is turned off, the moment remains at rest in the rotating frame, and hence precesses in the laboratory frame in a plane normal to the field. This suggests a way of observing magnetic resonance. Put the sample in a solenoid. Pulse the solenoid with an rf field that tips the nuclear spins by 90^o. Then as the spins precess in the x-y plane, they produce a time-varying flux through the coil that induces an observable emf according to Faraday's law.

coil.eps

What we have suggested so far would indicate that the induced emf would persist indefinitely, but in practice, the interactions of the spins with their surroundings cause a decay. Let $\tau$ denote the relaxation time. Then $\tau\sim$ milliseconds in liquids and $\tau\sim 100\;\mu$s in solids. Even during that short time, there are many precession periods. What we have described is the observation of the ``free induction decay'' (i.e., free of H₁).

T1decay.eps

We have been considering a uniform field $H_o\hat{z}$. To give the system some spatial resolution, one can vary the static field spatially by putting on a field gradient. Then only those spins that are located where $\omega=\gamma H_o$ will give a strong signal.

Spin Echoes

One can use clever combinations of signals to get information from NMR. Spin echoes were discovered by Erwin Hahn shortly after he finished his graduate studies. A $\pi/2$ pulse followed by a $\pi$ pulse a time $\tau$ later leads to an echo generated by the system at time $2\tau$.

spinecho.eps

If the spins initially are aligned along the z axis, the $\pi/2$ pulse puts them in the x-y plane where they dephase due to field inhomogeneities and spin-spin interactions. The $\pi$ pulse refocuses the spins and they produce an echo a time $2\tau$ later.

rephase.eps